How To Find APR From Ear

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for LiveWell, at no extra cost. Learn more)

Introduction

Understanding the Relationship Between APR and EAR

When it comes to managing personal finances or evaluating loan options, understanding the concepts of Annual Percentage Rate (APR) and Effective Annual Rate (EAR) is crucial. These two metrics play a significant role in determining the actual cost of borrowing or the potential return on investment. While APR represents the annual cost of borrowing, including fees and interest, EAR provides a more comprehensive measure by accounting for the effects of compounding. This article aims to demystify the relationship between APR and EAR and guide you through the process of calculating APR from EAR.

By gaining a clear understanding of these concepts, individuals can make informed decisions regarding loans, credit cards, and investment opportunities. Whether you're planning to take out a loan or comparing different financial products, being able to calculate APR from EAR empowers you to assess the true cost of borrowing and make well-informed financial choices. Let's delve into the intricacies of APR and EAR, unravel their connection, and equip you with the knowledge to navigate the realm of personal finance with confidence.

Understanding the Relationship Between APR and EAR

Annual Percentage Rate (APR) and Effective Annual Rate (EAR) are fundamental concepts in the realm of finance, particularly when it comes to borrowing and investing. APR represents the annual cost of borrowing, expressed as a percentage that includes not only the interest rate but also any additional fees or charges associated with the loan or credit card. On the other hand, Effective Annual Rate (EAR) takes into account the impact of compounding, providing a more accurate reflection of the true annual cost of borrowing or the actual annual return on investment.

It’s important to recognize that while APR is relatively straightforward to calculate, it doesn’t consider the effects of compounding, which can significantly impact the actual cost of borrowing. In contrast, EAR considers the frequency of compounding, providing a more realistic representation of the annual cost of borrowing or the annual return on investment. By understanding the relationship between these two metrics, individuals can gain insights into the true cost of borrowing and make informed decisions when evaluating loan options or investment opportunities.

When comparing different loan offers or credit cards, knowing how to calculate APR from EAR enables individuals to make direct comparisons, ensuring that they are fully aware of the actual cost of borrowing. This knowledge empowers consumers to select the most suitable financial products based on their individual needs and financial circumstances. Additionally, for investors, understanding the relationship between APR and EAR is essential for evaluating the potential returns on investment and making well-informed decisions when exploring various investment opportunities.

By comprehending the nuances of APR and EAR, individuals can navigate the complex landscape of personal finance with confidence, ensuring that they make informed decisions that align with their financial goals. In the following sections, we will delve into the process of calculating APR from EAR, providing practical insights and examples to further enhance your understanding of these critical financial concepts.

Calculating APR from EAR

Calculating the Annual Percentage Rate (APR) from the Effective Annual Rate (EAR) involves a straightforward yet essential process. Since EAR accounts for the impact of compounding, converting it to APR allows individuals to understand the annual cost of borrowing without the complexities of compounding frequency. The formula for this conversion is derived from the relationship between APR and EAR, providing a clear method for transforming the more comprehensive EAR into the widely used APR.

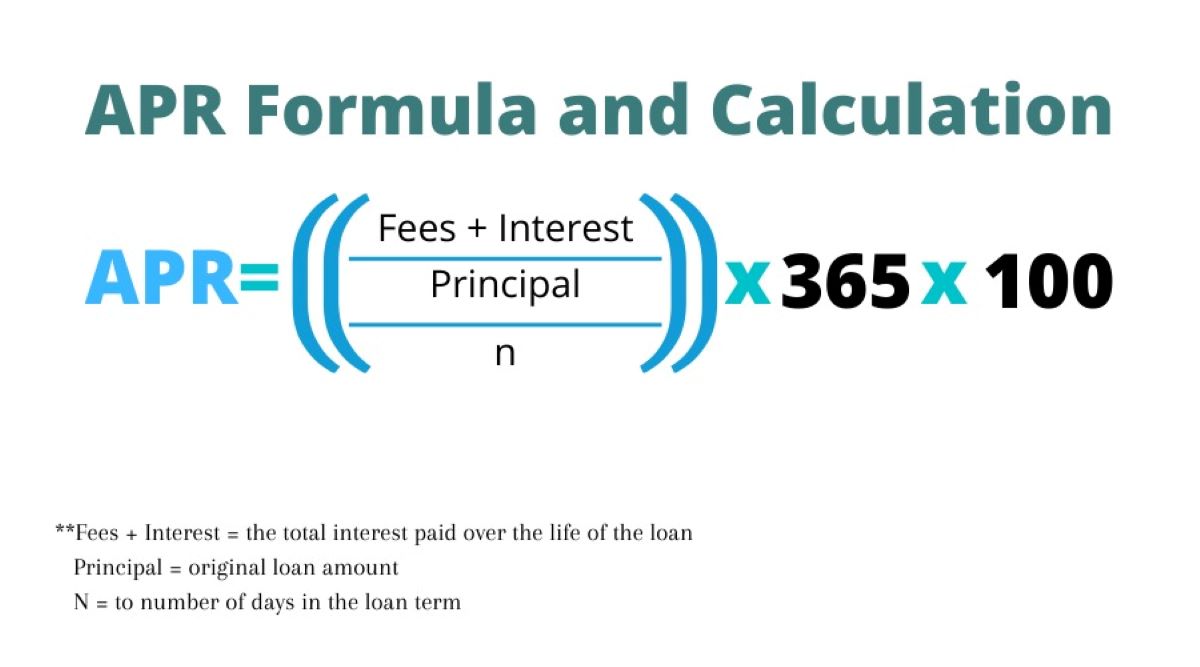
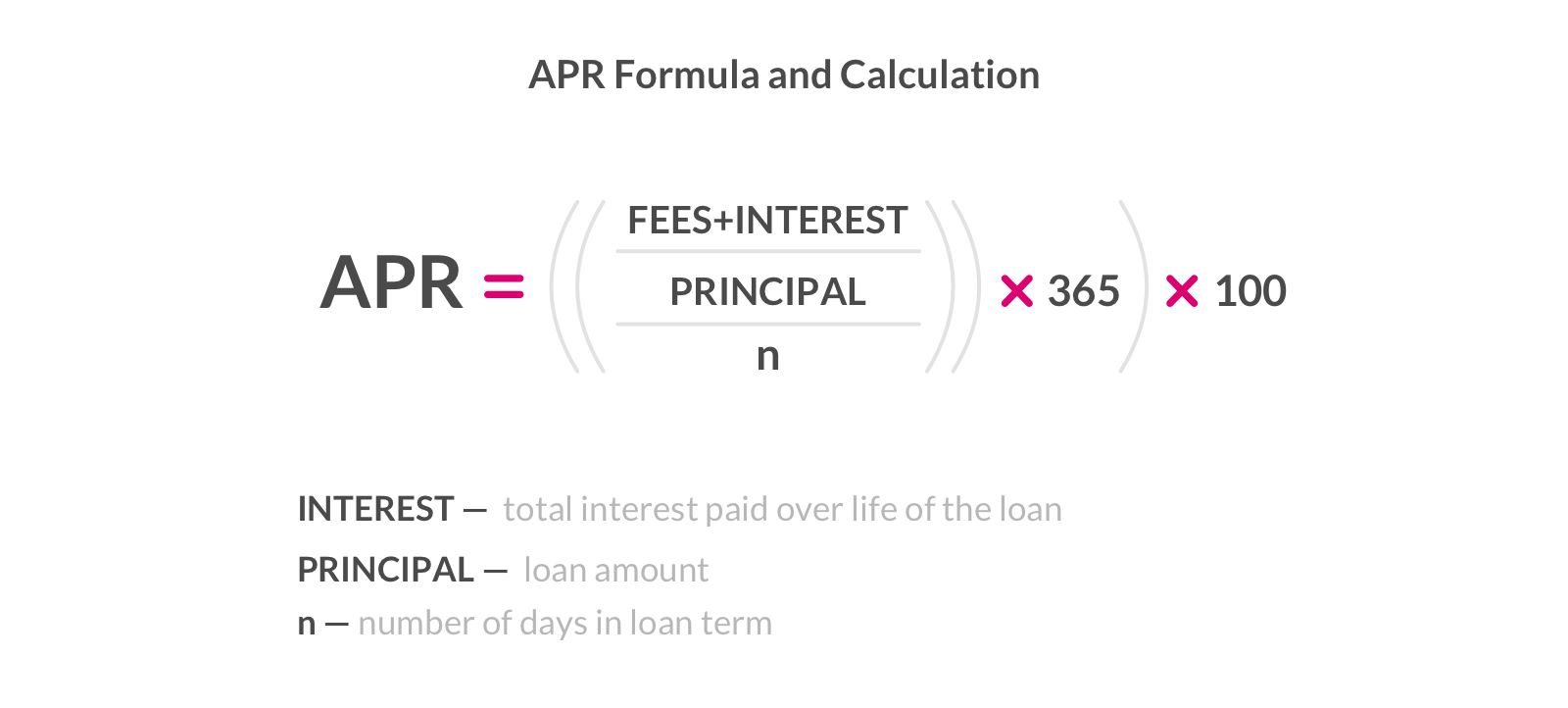
The formula for converting EAR to APR is as follows:

APR = (1 + (EAR/n))^n – 1

Where:
– APR represents the Annual Percentage Rate
– EAR denotes the Effective Annual Rate
– n signifies the number of compounding periods within a year

By applying this formula, individuals can obtain the APR from the given EAR, enabling them to compare the annual cost of borrowing across different loan options or credit cards effectively. Understanding this conversion process is invaluable for making informed financial decisions, as it provides a standardized metric for evaluating the true cost of borrowing, irrespective of the compounding frequency associated with the loan or investment.

It’s important to note that while the formula for calculating APR from EAR offers a simplified approach to understanding the annual cost of borrowing, it provides a reliable basis for direct comparisons between different financial products. This knowledge equips individuals with the tools to assess the actual cost of borrowing accurately, empowering them to select the most suitable loan or credit card based on their unique financial needs and preferences.

By mastering the process of converting EAR to APR, individuals can navigate the intricacies of personal finance with confidence, ensuring that they have a comprehensive understanding of the actual cost of borrowing and can make well-informed decisions when exploring various financial products.

Example of Calculating APR from EAR

To illustrate the process of calculating the Annual Percentage Rate (APR) from the Effective Annual Rate (EAR), let’s consider a hypothetical scenario. Suppose you are evaluating two loan options, each with a different compounding frequency and corresponding EAR. Loan A offers an EAR of 5.25%, compounded semi-annually, while Loan B presents an EAR of 5.5%, compounded quarterly.

Using the formula for converting EAR to APR, we can determine the APR for each loan option:

For Loan A:
APR = (1 + (0.0525/2))^2 – 1
APR ≈ (1 + 0.02625)^2 – 1
APR ≈ (1.02625)^2 – 1
APR ≈ 1.053144 – 1
APR ≈ 0.053144 or 5.31%

For Loan B:
APR = (1 + (0.055/4))^4 – 1
APR ≈ (1 + 0.01375)^4 – 1
APR ≈ (1.01375)^4 – 1
APR ≈ 1.056729 – 1
APR ≈ 0.056729 or 5.67%

Based on these calculations, we find that Loan A has an APR of approximately 5.31%, while Loan B has an APR of around 5.67%. This example demonstrates how the conversion from EAR to APR allows for a direct comparison of the annual cost of borrowing, enabling individuals to make informed decisions when selecting a loan based on their unique financial circumstances and preferences.

By understanding the process of calculating APR from EAR and applying it to practical examples, individuals can gain a deeper appreciation for the significance of these financial metrics and their impact on borrowing costs. Armed with this knowledge, individuals can confidently navigate the realm of personal finance, ensuring that they are equipped to make well-informed decisions when evaluating loan options and credit cards.

Conclusion

In conclusion, the relationship between Annual Percentage Rate (APR) and Effective Annual Rate (EAR) forms the cornerstone of understanding the true cost of borrowing and the potential returns on investment. By comprehending the nuances of these financial metrics and mastering the process of calculating APR from EAR, individuals can make informed decisions when exploring loan options, credit cards, and investment opportunities.

Understanding the impact of compounding and the conversion from EAR to APR empowers individuals to compare the annual cost of borrowing across different financial products, ensuring that they can select the most suitable options based on their unique financial needs and preferences. Whether evaluating loan offers or assessing potential investment returns, the ability to calculate APR from EAR provides a standardized method for determining the actual annual cost of borrowing, transcending the complexities of compounding frequencies.

Armed with this knowledge, individuals can navigate the complex landscape of personal finance with confidence, ensuring that they are equipped to make well-informed decisions that align with their financial goals. By unraveling the intricacies of APR and EAR and applying practical examples, this article has provided valuable insights into these critical financial concepts, equipping readers with the tools to make informed financial choices.

Ultimately, the ability to calculate APR from EAR represents a pivotal skill in the realm of personal finance, enabling individuals to assess the true cost of borrowing and make informed decisions that have a lasting impact on their financial well-being. By embracing this knowledge, individuals can embark on their financial journeys with clarity and confidence, equipped to navigate the complexities of borrowing and investing while making choices that align with their long-term financial objectives.